Sklansky Poker Hands

1. Sklansky Poker Hands
2. Sklansky Poker Hand Rankings
3. Sklansky Poker Starting Hands

Based on the tabulated data and chart generated, there are a few interesting observations to be made. The are listed below:

Sklansky hand groups was formulated by David Sklansky and Mason Malmuth. Both of these old school poker players understand the math very well. It is no surprise that our hand rankings aligns very well with their proposed hand groups. Sklansky hand group proposes that Tier 1 group consists of pair A, pair K, pair Q, pair J and suited AK. Synopsis of the Book Hold'em Poker. Updated for today's double blind structure. Contains the most up-to-date Sklansky Hand Rankings available. 1997 Edition Second Printing 2001 This is the first Hold'em book you should read before playing in any public poker room.-videopro Hold'em Poker by David Sklansky is must reading for anyone planning to play in Nevada, California, or any place else where.

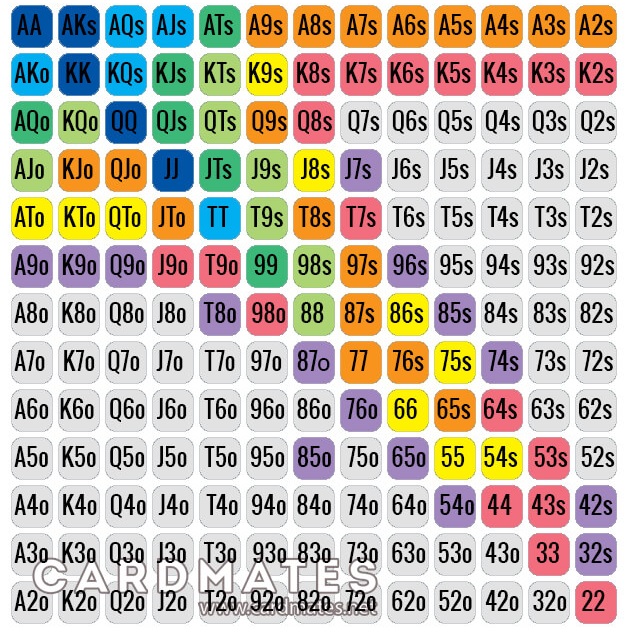
1. Pair A is best hand

There should be no surprise that Pair A is the best hand. Having a pair A, helps you to easily get the best possible double pair combo or three-of-kind combo. While it might be harder to strike straight or flush with it, those scenarios are typically less likely to happen. Thus, making pair A better in general.

2. Offsuit 72 is the worst hand

This might be less known to people and it could be counter intuitive. Some might have thought that perhaps Offsuit J2 would be worse than Offsuit 72. But, that is not the case.

Sklansky's Tournament Poker for Advanced Players is a book for those who already know a thing or two about poker but are looking to improve their tournament play specifically. In the book, Sklansky explains the biggest differences between cash games and tournaments and presents strategies you’ll need to adopt for a successful transition.

To understand why this is the case, we can start thinking about what are combinations that are most likely to lead to a winning combo assuming no one folds. Given any hands, we are more likely to win with double pair, followed by 3-of-a-kind, straight flush and so forth.

With offsuit 72, we are more likely to win double pair of pair 7 and pair 2, followed by three-of-a-kind and so on. However, it is also worthwhile to note that it is highly like other players has a better double pair or three-of-a-kind. This bring us to the next important lesson to learn.

3. Having a suited, closely connected hand with A, K or Q is better than having pairs that is less than 9

If you were to investigate the table or chart, the hand ranked 5th is Suited AK. What is even more interesting is pairs hand only took 6 spots from rank 1 to rank 20. Most of the remaining spots were taken up by suited, closely connected hands with a high card like A, K or Q.

The reason for this is similar to previous point that we made. It is more frequent that players will win using double pairs or 3-of-a-kind. Therefore, having a higher card helps to push you to a better standing to win.

One final note on this topic - Pair 9 is the last pair hand ranked in the top 20 hands. Playing any other pairs hand may not be as good as conventional wisdom might suggest.

4. Winning chance drops fast within the top 7 ranked hands

This is the lesson that really took us by surprised. While developing our poker odds calculator, we did had a sense that odds of winning was somewhat asymmetric. But, the chart above really solidify how much the asymmetry was.

Within the top 7 ranked hands, the probability of winning drops really fast from paired A to paired K and so forth. If you get the top 7 hands, you really should work hard to get through the preflop.

5. You are more likely to win a 6 players match than a 9 players one

A player with hands that are in the top 7 ranks in a 6 players game have a much better chance of winning in a 9 players game. For example, Paired A has roughly 49.5% preflop winning probability in a 6-player game compared to only 35% in a 9-players game. While 14.5% difference is not as big as it sounds, it has a significant impact on the pot odds that you will need to make a value play. In short, it might be easier to make money off a 6 players game rather than a 9 players game.

6. In a no-folding six players match, your hand range to play is very large

This point is not as crucial as other points we have made. But, we find this observation quite interesting although it is unlikely to happen in real life.

Suppose that we are in a no-folding 6 players Texas Hold'Em match. During every betting session, our pot odds is 5-to-1. This means that for every $1 we bet, we stand to win $5.

Based on this pot odds, our break-even pot equity or winning odds is around 16.67%. Using the chart above, we can see that we can play any hands better than rank 106. This means that players can play 105 types of hands out of 169 types (59.2% of all types) and still perform better than break even! Basically, you have a very large hand range to play in this type of situation.

Nonetheless, this is a just-for-fun analysis, which does not happen that often in real life. Based on some of our experience playing, it could happen sometimes during preflop though.

7. Our hand rankings are similar to Sklansky hand groups

Sklansky hand groups was formulated by David Sklansky and Mason Malmuth. Both of these old school poker players understand the math very well. It is no surprise that our hand rankings aligns very well with their proposed hand groups.

Sklansky hand group proposes that Tier 1 group consists of pair A, pair K, pair Q, pair J and suited AK. These cards are essentially ranked 1 to 5 via our Monte Carlo simulation. The same observation can be made for Sklansky Tier 2 and Tier 3 hand group.

Editor’s Note: This article was first published in the March, 2014 issue.

In part one, we looked at Norman Zadeh’s approximate game theory optimal solution to a heads up, pot-limit, one round poker game. Both players ante $1, the first player checks or bets $2 and if he checks, the second player bets either $2 or checks also. There are no raises.

Zadeh worked out that the first player should bet his top 14% and bluff with his bottom 7%. He should check and call with hands between 14 and 50 and check and fold with hands between 50 and 93. The second player should call with the top half of his hands and if checked to bet the top 30% and bluff with the bottom 15%. He would fold hands 50-85.

When both players use Zadeh’s strategy, the first player has an EV of about -8.5 cents.

What if one of the players differs substantially from this approximant game theory optimal strategy? To give you an idea of what happens, take a look at the following example. Suppose the first player uses the strategy where he bets the top 40% and the bottom 32% of his hands and checks and calls with the remaining hands, which are between 40 and 68. Against him, would player two’s GTO strategy, as specified by Zadeh, still work? Certainly, that strategy is far from the best when facing player one. For instance, player two should never bluff against player one since he will always be called. Secondly, when player one bets, the Zadeh strategy for player two will have him folding far too often. Finally, if player one checks, player two should value bet way more than his top 30%.

In spite of all these flaws, mathematicians contend that GTO does at least as well against bad players as it does against other GTO players. Thus, they would predict that sticking with Zadeh’s strategy will win player two at least 8.5 cents per hand. We will see if that’s right in part three of this essay.

Sklansky Poker Hands

For now, however, I think it would be a good exercise to come up with the perfect counter strategy to player one given that you know his strategy. Again, his strategy is to bet hand 0-40 and 68-100 and to check and call with the rest. Before reading further, see if you can do this yourself.

Suppose player one checks, how many hands can player two bet for value? Well certainly, he can bet 0-40, as they are all certain to win. But, he can also bet another 14% and still be the favorite. Do you see why? Player two will be calling from 40-68. If player one bets, we need to call with hands that have a better than 1/3 chance of winning since we are getting 2:1 odds. Notice that hand 68 is an easy call since it loses to hands 0-40, but beats the bottom 32% that he also bets. The fact is that player two can call with hands all the way down to 76. With that hand, he will lose to all of player one’s value bets and even to some of player one’s “bluffs”. Still though, he is only a 48:24 underdog.

We will now use the method I showed you in part one to calculate the EV of player one when facing the perfect counter strategy.

● If player one has hand 0-40 and player two has hand 0-40, there will be a bet and a call and they will break even. This happens 16% of the time and the EV is 0.

● If player one has hand 0-40 and player two has hand 40-76, there will be a bet and a call and player one will win $3. This will happen 14.4% of the time and the EV is 43.2 cents.

● If player one has hand 0-40 and player two has hand 76-100, there will be a bet and a fold and player one will win $1. This will happen 9.6% of the time and the EV is 9.6 cents.

● If player one has hand 40-54 and player two has hand 0-40, there will be a check and a call and player one will lose $3. This will happen 5.6% of the time and the EV is -16.8 cents.

● If player one has hand 40-54 and player two has hand 40-54, there will be a check and a call and they will break out even. This will happen 1.96% of the time and the EV is 0.

● If player one has hand 40-54 and player two has hand 54-100, it will go check-check and player one will win $1. This will happen 6.44% of the time and the EV is 6.44 cents.

● If player one has hand 54-68 and player two has hand 0-54, it will go check-call and player one will lose $3. This will happen 7.56% of the time and player one will lose 22.68 cents.

● If player one has hand 54-68 and player two has hand 54-68, it will go check-check and they will break even. This will happen 1.96% of the time and the EV is 0.

● If player one has 54-68 and player two has 68-100, it will go check-check and player one will win $1. This will happen 4.48% of the time and the EV is 4.48 cents.

● If player one has hand 68-76 and player two has hand 0-68, it will go bluff-call and player one will lose $3. This will happen 5.44% of the time and the EV is -16.32 cents.

● If player one has 68-76 and player two has 68-76, it will go bluff-call and they will break even. This will happen .64% of the time and the EV is 0.

Sklansky Poker Hand Rankings

● If player one has 68-76 and player two has 76-100, it will go bluff-fold and player one will win $1. This will happen 1.92% of the time and the EV is 1.92 cents.

● If player one has 76-100 and player two has 0-76, it will go bluff-call and player one will lose $3. This will happen 18.24% of the time and the EV is -54.72 cents.

● If player one has 76-100 and player two has 76-100, it will go bluff-fold and player one will win $1. This will happen 5.76% of the time and the EV is 5.76 cents.

When you add up player one’s EVs, it comes to -39.12 cents, much worse than how he would do if he stuck to his GTO strategy, even against a world class player.

To be continued…..

Sklansky Poker Starting Hands